噪声实验装置

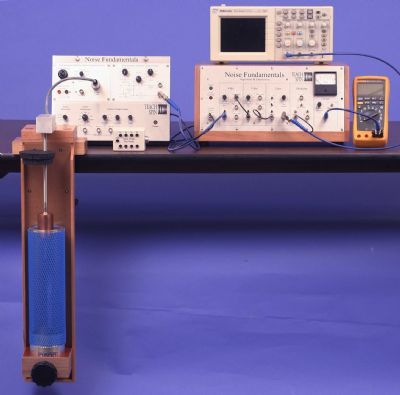
• 约翰逊噪声，“布朗运动”的电子检测和量化
• 演绎玻尔兹曼常数，K _乙，从约翰逊噪声对温度的依赖性
• 观察散粒噪声和量化，以衡量基本电荷ê '
• 用于多种测量的前端低一级的电路配置
• 调查的功率谱密度和电压噪声密度信号，他们的V / Hz和V /√Hz为单位
• 应用傅立叶噪声密度噪声信号转换成数字化处理的方法
• 探索放大，滤波的频率，平方和平均时间
• 培养技能适用于整个测量科学的广度

噪音基本装置是一组工具，先进的和中间的实验室教学和学习有关电子噪声。在所有的电子信号中存在的噪声限制了许多测量的灵敏度。这，本身将是足够的理由来激励学习如何可以量化噪声。但是，电子噪音可以远远超过滋扰，或限制-兰道尔的一句名言，有时噪音的信号“。事实上，至少有两种情况，即噪声的测量可以得到的基本常数。

通过集中处理和约翰逊噪声和散粒噪声测量，TeachSpin的噪声基础，让学生以确定双方玻尔兹曼常数，_K B ，电子电荷的幅度，' E '。

约翰逊噪声的波动电动势在任何**温度Ţ > 0 ​​电阻油然而生。Nyquist的预测是，这个电动势的均方服从< V ²（t）的> = 4 K _BTR D F ，D F 噪声测量带宽。此结果使要测量的玻尔兹曼常数 k _乙。

散粒噪声是衡量观察到的波动一定的电流，由于量化地对它们所加的粒度。在这种情况下，肖特的预测是一个直流电流我_直流服从另一均方有关的波动会伴随着，[δ 我（吨）] ² > = 2 ë键我_直流 D F。此结果使要测量的基本电荷ë键的大小。

从这些来源之一，还有更多的噪音，可以观察到，因为模块化，用户可配置的，我们的电子安排。本装置的信号流的透明布局使得它很清楚如何在实践中量化噪声，由于过程的放大，滤波的频率，平方和平均的时间，可以单独和详细理解。其结果是量化噪声的方法，和噪声密度，学生其实可以理解。这会产生一个便携式的一套适用于整个测量科学的广度技能。 噪声测量在物理学的许多领域中有一个非常当前的应用程序。

Introduction

• Detect and quantify Johnson noise， the ‘Brownian motion’ of electrons
• Deduce Boltzmann’s constant， k_B， from the temperature dependence of Johnson Noise
• Observe and quantify shot noise in order to measure the fundamental charge ‘e’
• Configure front-end low-level electronics for a variety of measurements
• Investigate ‘power spectral density’ and ‘voltage noise density’ of signals， and their V²/Hz and V/√Hz units
• Apply Fourier methods to digitally process noise signals into noise densities
• Explore amplification， filtering-in-frequency， squaring， and averaging-in-time
• Develop skills applicable across the breadth of measurement science

TeachSpin's Noise Fundamentals is a set of tools for teaching and learning about electronic noise in the advanced and intermediate laboratory. The noise present in all electronic signals limits the sensitivity of many measurements. That， in itself， would be reason enough to motivate learning how noise can be quantified. But electronic noise can be much more than a nuisance， or a limit -- in the famous phrase of Landauer， sometimes ‘noise is the signal’. In fact， there are at least two cases in which the measurement of noise can give the values of fundamental constants.

By concentrating on the processing and measurement of Johnson noise and shot noise， TeachSpin’s Noise Fundamentals allows students to determine both Boltzmann’s constant， k_B， and the magnitude of the charge on the electron， ‘e’.

Johnson noise is the fluctuating emf which arises spontaneously in any resistor at absolute temperature T > 0. Nyquist’s prediction is that the mean square of this emf obeys < V²(t) > = 4 k_B T R Df， where Df is the bandwidth over which noise is measured. This result allows Boltzmann’s constant k_B to be measured.

Shot noise is a measure of the fluctuations observed in certain currents， due to the granularity imposed on them by the quantization of charge. In this case， Schott’s prediction is that a dc current i_dc will be accompanied by fluctuations obeying another mean-square relation， < [δi(t)]²> = 2e i_dc Df. This result allows the magnitude of the fundamental charge e to be measured.

Noise from either of these sources， and many more， can be observed because of the modular， and user-configurable， arrangement of our electronics. The transparent signal-flow layout of our apparatus makes it very clear how noise is quantified in practice， since the processes of amplification， filtering-in-frequency， squaring， and averaging-in-time can be understood separately and in detail. The result is a method for quantifying noise， and noise densities， that students can actually understand. This generates a portable set of skills applicable across the breadth of measurement science.